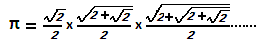Can the spirit of mathematics be mechanised? asks S.Ananthanarayanan.
Computers and ‘big data’ have transformed the nature of research in the sciences. Even in the arts, musicians, graphists and architects rely on computerised support. The mechanised input, however, is in the nature of ‘ support ’. The insight that is needed to sift data and see hidden patterns, or to add the creative touch to a composition, remains with the scientist or the artist.
Gal Raayoni, Shahar Gottlieb, Yahel Manor, George Pisha, Yoav Harris, Uri Mendlovic, Doron Haviv, Yaron Hadad and Ido Kaminer, from Technion–Israel Institute of Technology, and the Technion Harry and Lou Stern Family Science and Technology Youth Center, Haifa, in a paper in the journal, Nature, describe a possible way to automate even this genius, of the mathematician. They describe a method for computers to discover, from a mass of numbers, relationships that fit into formulae – to form the starting point of research into study of how these formulae arise and work.
Over the centuries, gifted mathematicians have identified cases of special numbers which have remarkable properties. And these properties, in turn, reveal more relationships and features, and then, could lead to applications. A well-known example is the number,Π (pi), which is the ratio of the circumference of a circle to its diameter. While this ratio is usually known as 22/7, or 3.14, this value is approximate. A better value is 3.14159, and even better is 3.141592653589793238. But the special feature of pi is that the ratio can never be exactly stated - not because of limitations of measurement, but for reasons of its very nature. Archimedes is credited with an early method, where many sided straight line figures (polygons) are drawn touching the inside and the outside of the circle. By increasing the number of sides, the shapes get closer to the circle itself, and the circumference can be approximated. And always improved by adding sides to the polygon!
Similar to the case of pi, we have another number, known as ‘e’, which is related to things that grow like a deposit that bears compound interest. Now, if the interest on the deposit is applied not once a year, but ever;six months, the interest accumulates a little faster. We can keep reducing the intervals of interest, to once a month, every day, every second, and so on. When the interval is very nearly zero, we find that in the time the deposit would normally have doubled, it reaches 2.71 times its value. This number, again, is not exact, a better value is 2.718281, and so on, and the exact value can never be worked out.
The number, ‘e’, has found application in many areas of science and engineering. One such is how fast the radioactivity of a sample would fall, while the number of atoms that are yet to decay keeps reducing. And in other areas, like working with waves, radio wave, light or lasers, atomic and nuclear science, biology, and lately, epidemiology, and the concept of ‘e’ has become fundamental.
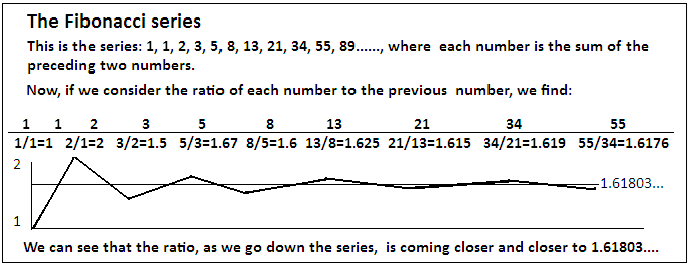
Yet another ratio, of the kind of ‘pi’ or ‘e’ is the ‘golden ratio’, which is the ratio of successive numbers in a series where each number is the sum of the previous two numbers. As we can see in the picture, this ratio progresses to be nearly 1.61803..., but can never be exactly evaluated. This, again, is a ratio that is found in leaves, shells, and many other places in nature.
Mathematicians, who had understood the way of numbers, experimented to discover these and other numbers and then formulae and expressions for calculating them.
Pi, for instance is given by:
Many of the relations were, at first, just conjectures, later verified and finally proved. One such is the celebrated Fermat‘s theorem of 1637, which says that the Pythagoras relation, a2=b2+c2, can never be true if a, b and c are integers and raised to a power more than 2. The relation was laboriously verified, again lately with the help of computers, but it defied proof for nearing 4 centuries.
Ramanujan
It is by way of conjectures like these that many important advances in mathematics have come about. The mathematician, Srinivasa Ramanujan (1887-1919) was a prolific contributor – identifying a host of spectacular relations, theorems, mathematical results, even in his short working career. Ramanujan had not been trained in the formal – proposition–axioms–proof– tradition of mathematics, but was a dropout, yet passionate about mathematics. Many of the results he put down are hence abruptly stated, without the context, discussion or proof. Deciphering his diaries, verifying the startling results that he records, and working out the proofs has been fodder for generations of researchers and students.
The authors of the paper in Nature observe that the many fundamental constants, like pi or ‘e’ have become ubiquitous in the sciences, but mathematical formulas that demonstrate links among them are hard to come by. There is regular progress in mathematics and refinement of existing knowledge, but startling discoveries that set off new courses of research arise sporadically, as results of ingenuity or profound insight of mathematical greats, like Carl Friedrich Gauss or Ramanujan. The authors hence propose making use of algorithms and modern computing potential to scan the nature of different mathematical constants, like pi or ‘e’, and try to fit the data into schemes or formulas – which could then be verified and lead to research of how and why they work. A mechanized programme where “computer algorithms use numerical data to unveil mathematical structures, thus trying to replace the mathematical intuition of great mathematicians,” the paper says. As mathematical constants are common in different areas of science, discoveries in the field would have wide application, the paper says. Considering the approach as a rough equivalent of Deep Blue, the chess playing computer, doing as well as Garry Kasparov, the grand master, the authors christen their approach “the Ramanujan machine.”
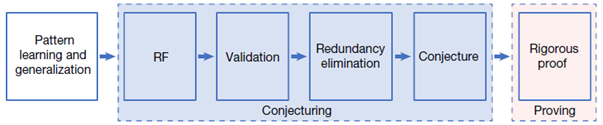
The method used is akin to using artificial intelligence to fit data to a hypothesis, usually a mathematical expression that can predict the future course of the data. The hypothesis is tweaked at each run of the program till it begins to fit the data. A similar approach, as shown in the diagram, is to propose, test and optimize a ‘regular formula’, denoted as ‘RF’, to arrive at a mathematical conjecture.
The authors report that algorithms have found dozens of well known formulas, as well as previously unknown ones. Some conjectures were simple to prove, some are yet to be proved, the paper says.
As huge computing resources are involved, the authors have followed the example of worldwide collaborative research programmes and launched a ‘Ramanujan machine’ project, where “the general community can donate computational time to find RFs, propose mathematical proofs for conjectured RFs, or suggest new algorithms for finding them,” the paper says.
------------------------------------------------------------------------------------------ Do respond to : response@simplescience.in-------------------------------------------