There are surprises that lurk in numbers, says S.Ananthanarayanan.
“Mathematics is the language God uses to write the universe,” said Galilei Galileo. Galileo may have said this because of the mathematical precision he saw in the way the Heavenly bodies moved. And maybe this was the quality Galileo was looking for in the Heavens, and happily, he found it.
Numbers, in any case have been the core of the work of the greatest mathematicians. And it would be good news to many, even those mathematically challenged, that some intriguing properties of numbers are what Niranjan Ramakrishnan, IT practitioner and polymath, best known for his reflective book on the relevance of Mahatma Gandhi to the contemporary world, has included in much of his extensive blogging. Niranjan was combatting ALS (Lou Gehrig’s disease) since 2009. It was when he started losing his faculties that he took to writing, on a range of subjects, mathematical curiosities being one of them. And his writing has become topical, and many have been writing in, when he recently succumbed to ALS.


One of the ideas Niranjan speaks of is prime numbers, which are numbers that have no divisors, like the numbers 3, 5, 7, 23, 31. Niranjan notes that all prime numbers, apart from 1 and 3, have to be just one number removed from a multiple of the number, 6. Table 1 displays examples of the earliest primes, and some large ones too.
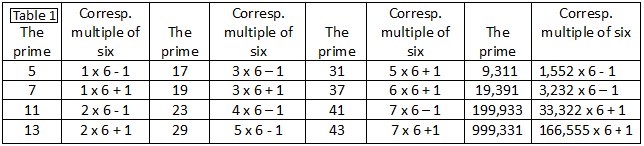
Niranjan goes on to show why this must be so. For starters, in any group of three consecutive numbers, example, 2, 3, 4 or 51, 52, 54 or 130, 131, 132, one of the numbers must be a multiple of 3. We can see that in the first group, we have the number, 3, in the second group, we have the number, 51 and in the third group, the number, 132. And we can see that this must be true for any three consecutive numbers. Now, if the group of three numbers has a prime as the middle number, for example, the prime, 11, in 10, 11, 12, the middle number can have no factors. And hence, one of the remaining two must be the multiple of 3. Again, as the middle number is a prime, it has to be an odd number. This implies that the other two numbers are even numbers. And, as an even number, the number that is a multiple of 3 is also a multiple of 2 x 3, which is 6. And hence, any prime has to come before or after a multiple of 6!
In one of his blogs, Niranjan noticed a pattern about the number 25 (and later found that it was there with other numbers too). Let us start with the squares of pairs of numbers evenly on either side of the number, 25. For example, 20 and 30, are on opposite sides of 25, and equally distant from 25. Other pairs are 21 and 29, or 22 and 28, and so on, as in Table 2. The first pair is 24 and 26, whose squares are 576 and 675. We can see that last two digits of the squares are the same (76) and the difference of the squares is 100. In the case of the second pair, 23 and 27, also, the last two digits of the squares are the same and they differ, this time, by 200. This pattern holds for other pairs of flanking numbers, down to 12 and 38, or 5 and 45, even negatives, -2 and 52, with squares of 4 and 2,704, differing by 2,700.
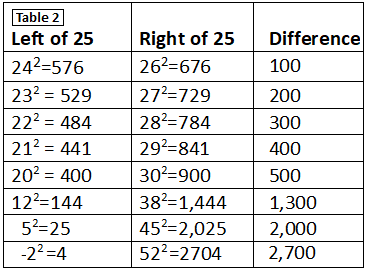
Niranjan goes on to note that this is a property of any number, with respect to flanking numbers at the same distance away from the centre, or the fulcrum, like the middle of a see-saw. And he works it out that the differences are in multiples of 100 because 25 x 4 = 100. If the fulcrum had been some other number, 27, for instance, the differences would have been multiples of 27 x 4 = 108. To try it out - if the fulcrum is 27, the first neighbouring pair is 26 and 28, and the squares are 676 and 784, which differ by 108. If we consider the pair, 25 and 29, the squares are 625 and 841, which differ by 216, and so on. “Now, it's easy to mentally reckon squares of numbers,” Niranjan’s blog says. For example, to get the square of 182, if we use 100 as the fulcrum, the partner number is 18. And 182 squared is 82 x 400 + 18 squared, or 32800 + 324, or 33124.
Another number that caught Niranjan’s fancy was Karpekar’s constant. This is the number, 6174, which arises from any four-digit number. The method is to take the four-digit number and arrange the four digits in ascending order, and then in descending order. For instance, if we start with 4728, we create two numbers, 8742 and 2478. Now, subtract the smaller from the larger. 8742 – 2478 is 6264. Now, repeat the procedure – the two numbers are 6642 and 2466, and the difference is 4176. When w repeat the procedure, 4176 leads to 1467 and 7641, resulting in 6174. And, now that we at 6174, we find that the procedure creates no other numbers, for 6174 leads to 1467 and 7641 and the difference, it is again 6174 (please see Figure 3, which has other starting numbers).

Now, this number, 6174, has other interesting properties. One of these is that it is divisible by the sum of its own digits: 6 +1 + 7 + 4 = 18. And 6174 = 18 x 343. Such a number, that can be divided by the sum of its digits, is called a Harshad number or a Niven number. And another property of 6174 is that it can be built up by the sum of powers of 18: 183 + 182 + 181 = 5832 + 324 + 18 = 6174. The discoverer, incidentally, of this and Harshad numbers, was D R Karpekar, a schoolmaster in Nashik, Maharashtra, who was a formidable mathematician. Niranjan, of course, would not rest after reading Karpekar, he went on to check if there were such numbers based on a 3-digit starter, and then to generalise to other starters!
As a practicing professional in the world of computer and software, this facility and fascination with numbers found ample expression.
But Niranjan’s interests were wide-ranging.
An important part of his work, different,
but not so far, from Gandhi and mathematics,
is the two volume set of Bantaism,
a compilation of Sardar Jokes – with sensitive
and philosophical interpretation of the genre,
as illustrative of the foibles of all of us.
------------------------------------------------------------------------------------------ Do respond to : response@simplescience.in-------------------------------------------